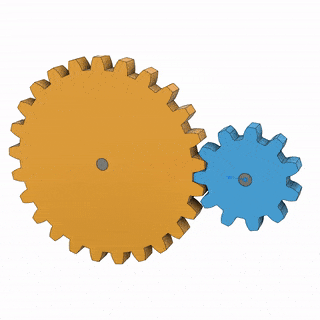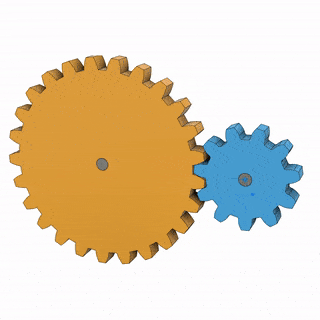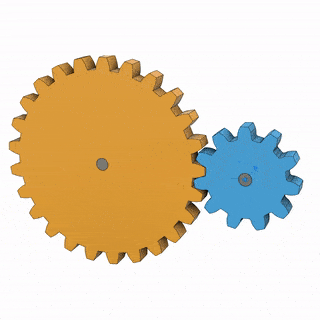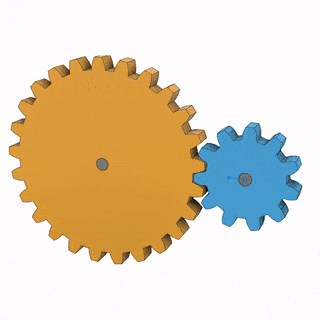
 Gears are used to change speed in rotational movement. In the example above the blue gear has eleven teeth and the orange gear has twenty five. To turn the orange gear one full turn the blue gear must turn 25/11 or 2.2727r turns.
Gears are used to change speed in rotational movement. In the example above the blue gear has eleven teeth and the orange gear has twenty five. To turn the orange gear one full turn the blue gear must turn 25/11 or 2.2727r turns.Notice that as the blue gear turns clockwise the orange gear turns anti-clockwise.
In the above example the number of teeth on the orange gear is not divisible by the number of teeth on the blue gear. This is deliberate. If the orange gear had thirty three teeth then every three turns of the blue gear the same teeth would mesh together which could cause excessive wear. By using none divisible numbers the same teeth mesh only every seventeen turns of the blue gear.
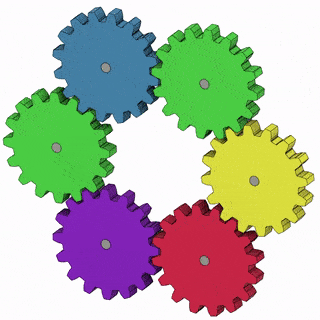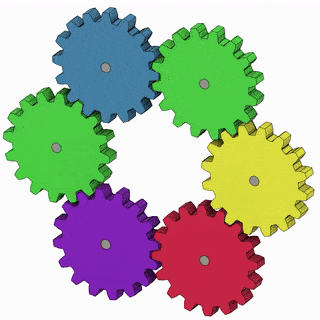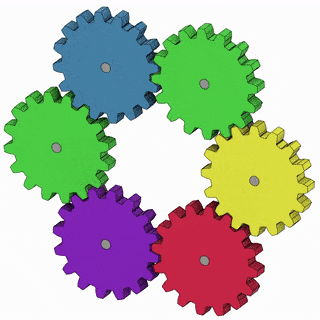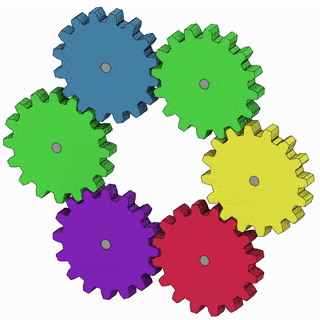 Rings of Gears and the Two Pound Coin
Rings of Gears and the Two Pound CoinGears turn in alternate directions. In the example, left the blue gear turns anti-clockwise, the two green gears on either side of it are then driven clockwise, these in turn drive the yellow and purple gears anti-clockwise and so on…
A ring of gears containing an even number of gears turns freely, as you can see, but imagine a ring with an odd number of gears , image that the blue gear is removed, you will see that the two green gears be turning the same direction and so would not be able to turn, the gears would lock together.
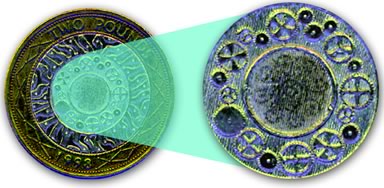
Now, look at the ring of gears around the centre
of the British two pound coin. Nineteen gears…!
Check out the gears model to download and make in the shop!



